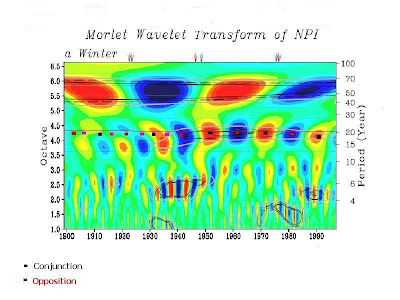
The diagram below shows a Morlet Wavelet Transform of the winter (North Pacific Index) NPI index.
The North Pacific (NP) Index is the area-weighted sea level pressure over the region 30°N-65°N, 160°E-140°W. The NP index is defined to measure interannual to decadal variations in the atmospheric circulation.
Reference: Shoshiro Minobe
GEOPHYSICAL RESEARCH LETTERS, VOL. 26, No. 7, Pages 855-858, APRIL, 1, 1999
Resonance in bidecadal and pentadecadal climate oscillations over the North Pacific: Role in climatic regime shifts
Superimposed on this plot are the times of Jupiter-Saturn Conjunction (when these two planets are on opposite sides of the Sun) which are spaced by 19.86 years, and the times of Jupiter-Saturn Opposition (when the two planets are aligned on the same side of the Sun), also spaced by 19.86 years.
This result is supported by a comparable figure from:
ADVANCES IN ATMOSPHERIC SCIENCES, VOL. 20, NO. 5, 2003, PP. 694–710 694
Joint Propagating Patterns of SST and SLP Anomalies in the
North Pacific on Bidecadal and Pentadecadal Timescales
ZHU Yimin and YANG Xiuqun
What we see in these figures is a remarkable match between the phase and period of Jupiter-Saturn conjunctions and oppositions and the bi-decade cycle in the winter NPI index. This match is best between the years of 1947 and 1991.
It is also apparent that groups of three bi-decadal cycle in the Winter NPI index are nested in phase inside a penta-decadal cycle of roughly 55 years in length. You can see one complete penta-decadal cycle starting with a Jupiter-Saturn opposition in 1922 and ending three Jupiter-Saturn oppositions later in 1981.
Amazingly, the Sun's motion about the Barycentre (i.e. center-of-mass) of the Solar System undergoes one orbital loop from one Jupiter-Saturn conjunction (or opposition) to the next every 19.86 years. Each orbital loop of the Sun about the Barycentre rotates by roughly 120 degrees with respect to the stars, compared to its previous orbital loop. Hence, it takes three orbital loops (i.e 3 x 19.86 = 59.6 years) for the Sun's Barycentric motion to rotate once with respect to the stars.
This means that solar inertial motion (SIM) about the Barycentre mimics the three bi-decadal cycles nested [in-phase] inside a longer penta-decadal cycle. The synchronization between these two phenomenon is quite remarkable and suggests that there may be an underlying physical link.

Found a similar set up with schwabe triplets
ReplyDeletehttp://weathercycles.wordpress.com/2014/05/15/schwabbe-triplets-and-earths-climate/
weathercycles (aka Susan Morrow).
ReplyDeleteThere is also a connection to the Moon’s tides and El Ninos that seems to match the dates you have given for the Schwabe triplets:
1913 to 1944 is schwabe triplet ( 15,16,17) warming
1944 to 1975 is schwabe triplet ( 18,19,20) cooling
1975 to 2008 is schwabe triplet ( 21, 2, 23) warming
If you see my work at: [sorry, it is pay-walled]
http://multi-science.atypon.com/doi/abs/10.1260/0958-305X.24.3-4.497
Wilson I. R. G., 2013, Are Global Mean Temperatures Significantly Affected by Long-Term Lunar Atmospheric Tides?, Energy and Environment, 24, Issue 3-4, pp. 497 – 508.
In this paper, I claim that the ratio of the frequency/intensity of El Nino’s to La Nina’s has a ~ 60 year pattern that almost precisely match the ~ 60 cooling and warming pattern of the Earth’s mean temperature. During the first ~ 30 years of this cycle the frequency/intensity of El Nino’s dominate that of the La Nina’s where you get warming of the World’s mean temperature. This is followed by a ~ 30 period where the frequency/intensity of La Nina’s dominate that of the El Nino’s which produces cooling.
I link the ~ 60 year cycle in the ratio of the frequency/intensity of El Nino’s to La Nina’s to the 31/62 year Perigee-Syzygy lunar tidal cycle.
A detailed investigation of the precise alignments between the lunar synodic [lunar phase] cycle and the 31/62 year Perigee-Syzygy cycle between 1865 and 2014 shows that it naturally breaks up five 31 year epochs each of which has a distinctly different tidal property. The first 31 year interval starts with the precise alignment on the 15th of April 1870 with the subsequent epoch boundaries occurring every 31 years after that:
Epoch 1 – 15th April 1870 to 18th April 1901
Epoch 2 – 18th April 1901 to 20th April 1932
Epoch 3 – 20th April 1932 to 23rd April 1963
Epoch 4 – 23rd April 1963 to 25th April 1994
Epoch 5 – 25th April 1994 to 27th April 2025
If you allow ~ 10 years lag for the effects of 31/62 year lunar tidal cycle to influence the frequency/intensity of El Nino’s to La Nina’s (mainly due to time it takes the ocean’s current to redistribute the excess heating in the tropics) then the boundaries between tidal epochs are shifted to:
Epochs 1 and 2: 1911
Epochs 2 and 3: 1942
Epochs 3 and 4: 1973
Epochs 4 and 5: 2004
which match in reasonably well with your Schwabe triplet years of 1913, 1944, 1975, and 2008.